Although weightlessness in space is an amazing experience, if humans stay in a weightless environment for a long time, their bodies will develop all kinds of problems, such as muscle atrophy, loss of bone density, etc. Therefore, if humans want to truly enter the starry sea, artificial gravity technology is necessary to do so.

How can artificial gravity be created?

The method of creating artificial gravity has been around for a long time. More than 100 years ago, tsiolkovsky, who is regarded as the father of spaceflight, suggested that we could use the "Centrifugal force" Generated by the rotation of an object to simulate gravity. It is important to note that "Centrifugal force" Is a virtual force introduced to facilitate the description of non-inertial reference systems and is essentially a manifestation of the inertia of the object.

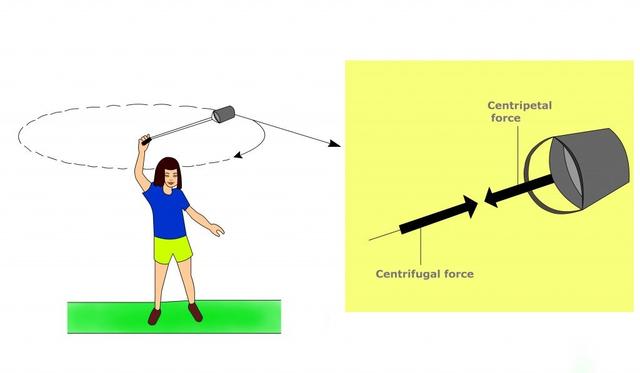
As shown in the diagram above, when we pull a bucket of water around ourselves with a string and rotate it at a certain speed, the water in the bucket will not spill, and this happens because the water in the bucket is subject to "Centrifugal force".

In the same way, if we make a space station in space in the shape of a ring and make it rotate, humans in it will feel the 'centrifugal force' pushing them towards the outer walls of the station, where they usually think of themselves as a reference system (i.e. In this case, the human would be able to stand firmly on the outer wall of the station, just as if he/she were feeling gravity.

In theory, it is perfectly feasible to use "Centrifugal forces" To simulate gravity, and such stations have been used in some science fiction works. However, in the real world, although humans have space stations, none of them are of this design.

Why don't space stations use artificial gravity?

For a space station to perfectly simulate the gravity of the earth's surface (i.e. Standard gravity) by rotating in space, it must equal the 'centrifugal force', which is described by the formula f=mg and the 'centrifugal force' by the formula f=mrω^2 where m represents the mass of the object, g represents the acceleration of gravity at the earth's surface (9.8m/s^2), r represents the radius of rotation, and ω represents the angular velocity.

The angular velocity can in turn be described by the formula ω = 2π/t, where t represents the time (in seconds) it takes for the rotating system to make one revolution. After a simple derivation, we can arrive at the formula: (9.8/r) = 2π/t under the root sign, which helps us to describe the relationship between the radius of rotation and the speed of rotation when the artificial gravity generated by the space station is equal to the gravity at the earth's surface.

For example, when r equals 10 metres, t equals 6.35 seconds, which means that all we need to do is build a space station with a 10 metre radius of rotation and have it rotate every 6.35 seconds to produce the effect of standard gravity. But is it really that simple? The answer is no.

The first problem is the gradient of artificial gravity. Since the magnitude of the "Centrifugal force" Is proportional to the radius of rotation, and humans are tall, the smaller the radius of rotation, the more pronounced the difference between the artificial gravity applied to the head and the feet. In addition to this, the impact of the "Coriolis force" On humans cannot be underestimated.

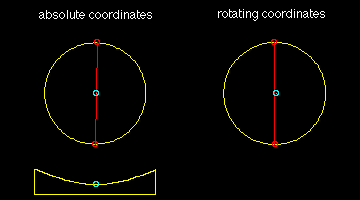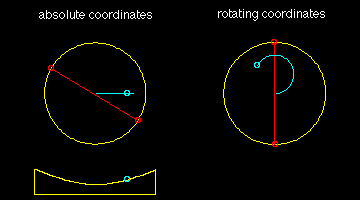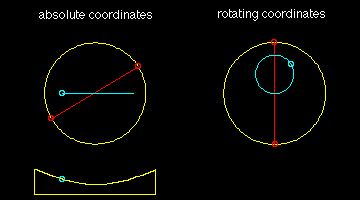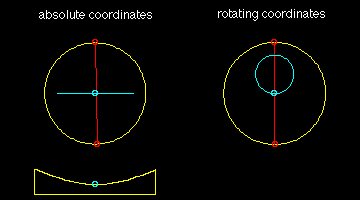
As shown in the diagram above, an object moving in a straight line in a rotating system has a tendency to continue in the same direction of motion due to inertia, but the rotating system itself is rotating, so if viewed from the perspective of the rotating system, the object does not move in a straight line, as if it were subject to some force.

This force is known as the "Coriolis force", but it should be noted that the "Coriolis force" Is still essentially a manifestation of the inertia of the object, so it is also a virtual force. It is clear that humans are affected by the coriolis force whenever they move through a rotating space station, and since its magnitude is proportional to the speed of rotation, the higher the speed of rotation, the greater the impact on humans.

This means that a station with too small a radius of rotation, or too fast a rotation speed, is not suitable for humans, who would suffer from dizziness, nausea and disorientation, or even coma if they were in such a station. So we must build a station with a large enough rotation radius and limit its rotation speed to what is acceptable to humans.

Studies have shown that the "Coriolis force" Has negligible impact on humans when the rotation speed is below 30 seconds per revolution, in which case, according to the previous formula, a rotation radius of approximately 224 metres is required to produce artificial gravity comparable to standard gravity.

From a technical point of view, such a space station using artificial gravity could be built. In the past, scientists have tried to design space stations using artificial gravity, but have finally given up, why? The reason is that the financial budget is too huge.

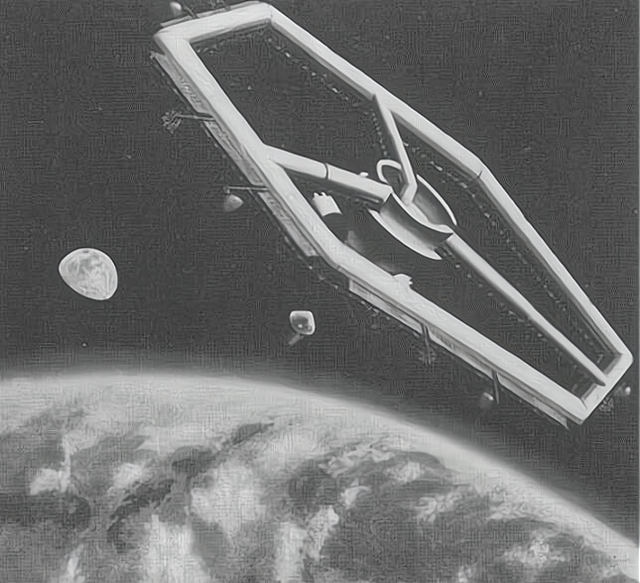
(the diagram shows nasa's proposed concept of a rotating space station back in 1962)

With the current level of human technology, it would cost at least us$4600 to launch an object with a mass of 1kg into space, and a giant space station with a radius of 224m would have a mass of at least 20,000 tonnes, so we would need to spend at least us$92 billion to launch this space station into space.

And the cost of developing, manufacturing and maintaining such a huge station would be very high, estimated to be in the hundreds of billions of dollars. Obviously, this is an unacceptable figure, and that is why the current station does not use artificial gravity.