According to known exploration data, the deepest seabed on earth is the marianas trench, located at the bottom of the pacific ocean. The measurement data shows that the deepest part of the marianas trench can reach 11,034 meters, where the pressure is about 110 mpa, equivalent to 1.1 tons of weight per square centimeter. So the question arises, if you take a 100kg solid iron ball and sink it into the deepest part of the sea floor, will the iron ball be deformed by the pressure?

In a three-dimensional space, the pressure on an object can come from three directions (x, y and z), so the pressure on an object can be classified as one-way pressure, two-way pressure and three-way pressure. It should be noted that the resistance to deformation of an object varies greatly between the different pressure cases, and is highest in the case of a three-way pressure.

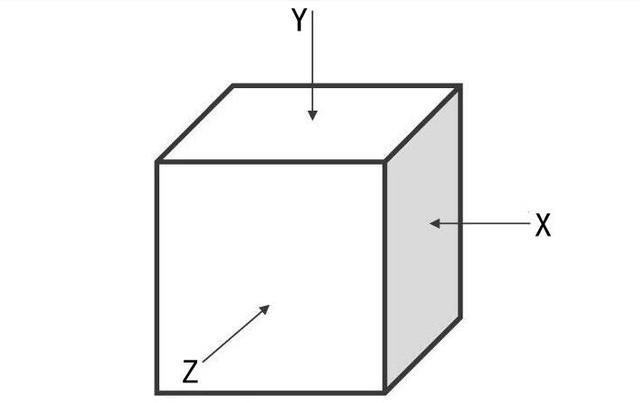
From a microscopic point of view, this phenomenon can be easily explained: An object can be considered as a large pile of atoms. In the case of three-way pressure, these atoms are not able to "Slip away", so their resistance to deformation becomes very high.
An object subjected to water pressure in seawater from all directions is typically a three-way pressure.
The density of iron is about 7.9 g/cm3, from which we can calculate that a solid iron ball of 100 kg has a diameter of about 23 cm, and in seawater the difference in pressure between its top and bottom is only about 2300 pa, a negligible value compared to the pressure in the deepest part of the earth's ocean floor (110 mpa).
So we can simply assume that the pressure on this solid iron ball in seawater from all directions is evenly distributed.

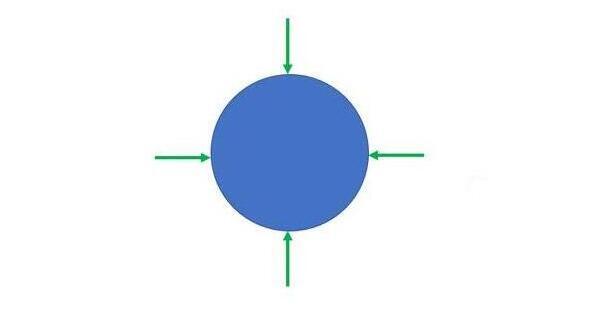
In this three-way pressure situation, the iron atoms that make up the solid iron sphere are "Inescapable" And therefore cannot be flattened directly as one would expect, but to deform it, one would need to compress the spaces between the iron atoms, in other words, to bring them closer together and thus make them shrink.
As we all know, an atom consists of a nucleus and electrons moving around it. Since the electrons are negatively charged, there is a repulsive force between the atoms, and the closer they are to each other, the greater this repulsive force becomes (the magnitude of the electromagnetic force is inversely proportional to the square of the distance).

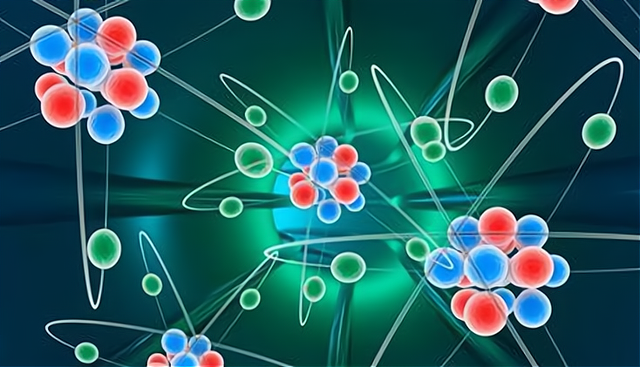
It can be seen that it is actually quite difficult to get the iron atoms closer together, in fact, even a pressure of 110 mpa can only compress the diameter of this iron ball a little bit (probably only a few tens of microns), and we cannot even observe this slight deformation with the naked eye.
So we conclude that if a 100kg solid iron ball is sunk to the deepest depths, it will indeed deform, but the deformation is so slight that we can usually ignore it. So is there a greater pressure on the earth that would deform this solid iron ball more significantly? The answer, of course, is yes.

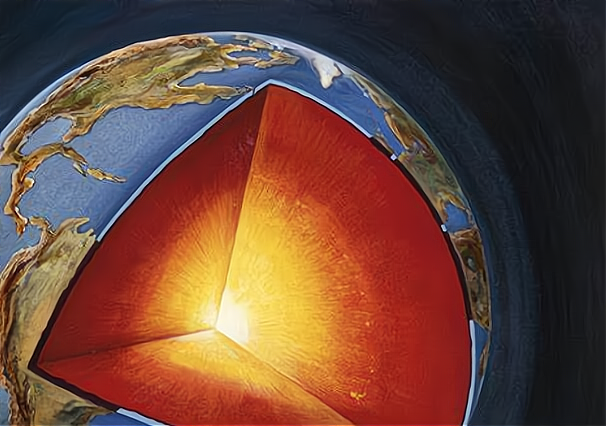
Our earth weighs 60 trillion billion tons and has a pressure at its core of 370 gpa, which is about 3364 times the pressure at the deepest ocean floor. In fact, the material in the earth's core is mainly elemental iron and nickel, while the average density of the core is 10.7 g/cm3. Obviously, if we were to place this solid iron ball in the core of the earth, the deformation would be very considerable.
It is worth noting that the pressure at the centre of the earth is nothing in the universe. The greater the mass of an object, the higher the pressure inside it, to the point where the pressure inside a massive object can even "Crush" Iron atoms, as is the case with neutron stars.

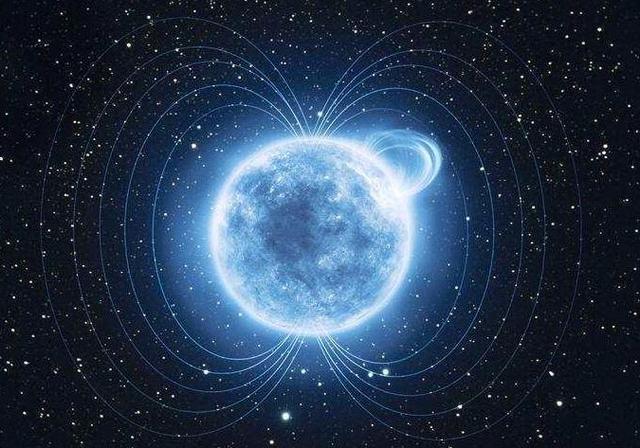
If we put an iron sphere inside a neutron star, the electrons in the iron atoms that make up the sphere would be pressed directly into the nucleus by the strong pressure and form neutrons with the protons in the nucleus. "Instead, it should be called a "Neutron sphere".
The diameter of an iron atom is of the order of 10^-10 metres, while the diameter of an iron nucleus is of the order of 10^-15 metres, which means that after the 100-pound solid iron ball is placed inside a neutron star, its diameter will shrink from 23 centimetres to 23 micrometres, and its volume will shrink by a factor of 1 trillion, and an object of this size will require a microscope to see.